Kunneth Formula

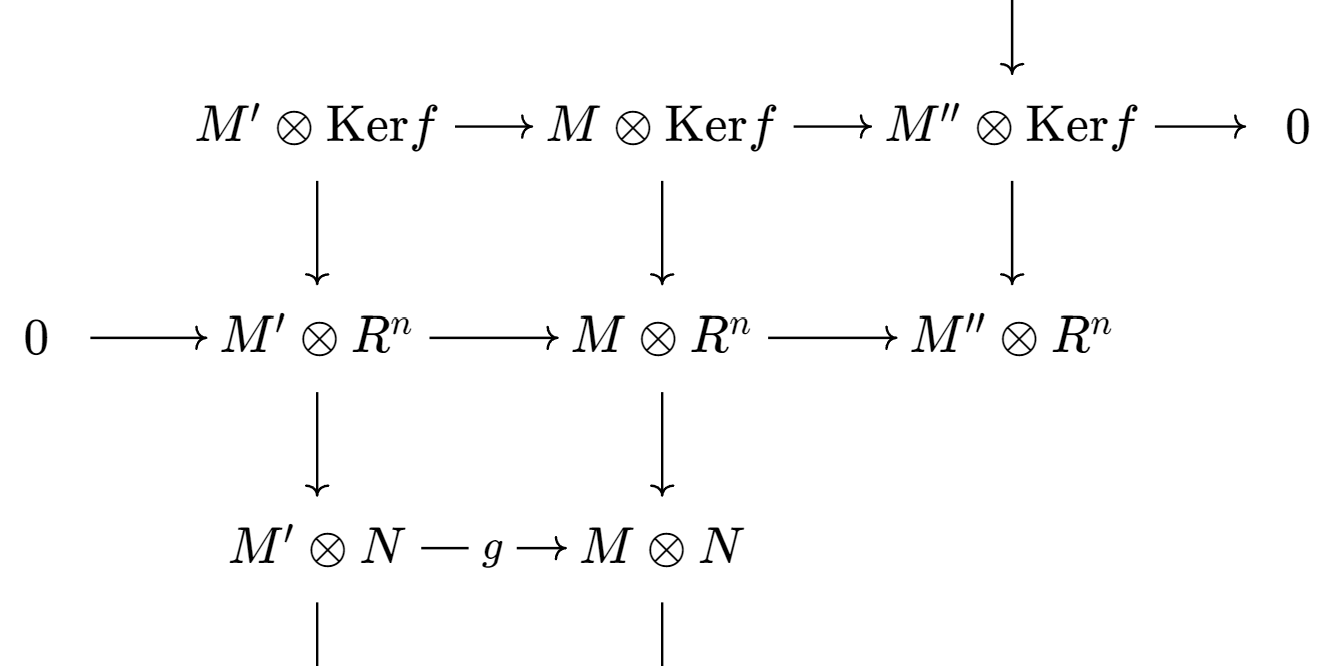
Proof. 1. We take a free presentation $R^{n}\xrightarrow{f} N$ of $N$ and get the exact sequence $0\to\mathrm{Ker}f\to R^{n}\to N\to 0$. Then we tensor the exact sequence $0\to M'\to M\to M''\to 0$ to it and obtain the following commutative diagram:

Each rows and columns are exact. By the snake lemma, $g$ is injective, thus $0\to M'\otimes N\to M\otimes N\to M''\otimes N\to 0$ exact.

2. Take an injection $f: N'\to N$ and tensor $0\to M'\to M\to M''\to 0$, we have the following diagram

Its rows exact by 1. If $M$ flat, $\alpha$ is injective thus $\alpha'$ injective, so $M'$ flat. If $M'$ flat, then $\alpha'$ is injective, from 5 lemma, $\alpha$ is injective, thus $M$ is flat. ◻

Proof. Notice that we have the exact sequence

$$0\to \mathrm{Ker}d_{n}\to P_{n}\to \mathrm{Im}d_{n}\to 0$$

Tensor $M$ on it, from the lemma above, we have exact sequence

$$0\to \mathrm{Ker}d_{n}\otimes M\to P_{n}\otimes M\to \mathrm{Im}d_{n}\otimes M\to 0$$

It induces a short exact sequence of chain complex

$$0\to \mathrm{Ker}d\otimes M\to P\otimes M\to\mathrm{Im}d\otimes M\to 0$$

Use the long exact sequence theorem, since $H_{n}(\mathrm{Ker}d\otimes M)=\mathrm{Ker}d_{n}\otimes M$, $H_{n}(\mathrm{Im}d\otimes M)=\mathrm{Im}d_{n}\otimes M$, we have the long exact sequence

$$\cdots\to \mathrm{Im}d_{n+1}\otimes M\to \mathrm{Ker}d_{n}\otimes M\xrightarrow{f}H_{n}(P\otimes M)\to \mathrm{Im}d_{n}\otimes M \xrightarrow{g}\mathrm{Ker}d_{n-1}\otimes M\to \cdots$$

For the short exact sequence

$$0\to \mathrm{Im}f\to H_{n}(P\otimes M)\to\mathrm{Ker}g\to 0$$

we compute $\mathrm{Im}f$ and $\mathrm{Ker}g$. From the snake lemma, we have the morphism $\mathrm{Im}d_{n+1}\otimes M\to \mathrm{Ker}d_{n}\otimes M$ is $\delta\otimes M$, where $\delta$ is the connecting morphism in the long exact sequence derived by $0\to \mathrm{Ker}d\to P\to \mathrm{Im}d \to 0$, i.e.

$$\cdots\to \mathrm{Im}d_{n+1}\xrightarrow{\delta}\mathrm{Ker}d_{n}\to H_{n}(P)\xrightarrow{0}\mathrm{Im}d_{n}\to \cdots$$

Since $-\otimes M$ is right exact, the diagram is commutative with exact rows

We have $\mathrm{Im}f\cong H_{n}(P)\otimes M$.

On the other hand, we consider the short exact sequence

$$0\to \mathrm{Im}d_{n}\to \mathrm{Ker}d_{n-1}\to H_{n-1}(P)\to 0$$

Using the long exact sequence theorem of $\mathrm{Tor}$

$$\cdots\to \mathrm{Tor}_{1}(\mathrm{Ker}d_{n-1},M)\to \mathrm{Tor}_{1}(H_{n-1}(P),M)\to \mathrm{Im}d_{n}\otimes M\to \mathrm{Ker}d_{n-1}\otimes M\to \cdots$$

To compute $\mathrm{Tor}_{1}(\mathrm{Ker}d_{n-1},M)$, we consider the short exact sequence

$$0\to \mathrm{Ker}d_{n}\to P_{n}\to \mathrm{Im}d_{n}\to 0$$

Using the long exact sequence theorem of $\mathrm{Tor}$

$$\cdots\to \mathrm{Tor}_{2}(\mathrm{Im}d_{n},M)\to \mathrm{Tor}_{1}(\mathrm{Ker}d_{n},M)\to \mathrm{Tor}_{1}(P_{n},B)\to \cdots$$

Since $\mathrm{Im}d_{n}$, $P_{n}$ flat, $\mathrm{Tor}_{k}(\mathrm{Im}d_{n},M), \mathrm{Tor}_{k}(P_{n},M)=0$. We have $\mathrm{Tor}_{1}(\mathrm{Ker}d_{n},M)=0$. Notice $\mathrm{Ker}d_{0}=P_{0}$, we finally have

$$0\to \mathrm{Tor}_{1}(H_{n-1}(P),M)\to \mathrm{Im}d_{n}\otimes M\xrightarrow{g}\mathrm{Ker}d_{n-1}\otimes M$$

So $\mathrm{Ker}g=\mathrm{Tor}_{1}(H_{n-1}(P),M)$. Finally

$$0\to H_{n}P\otimes M\to H_{n}(P\otimes M)\to \mathrm{Tor}_{1}(H_{n-1}(P),A)\to$$

◻

Proof. Notice that for PID $R$, the exact sequence

$$0\to\mathrm{Ker}d_{n}\to P_{n}\to \mathrm{Im}d_{n}\to 0$$

splits. After tensoring $M$, the exact sequence

$$0\to\mathrm{Ker}d_{n}\otimes N\to P_{n}\otimes N\to \mathrm{Im}d_{n}\otimes M\to 0$$

splits. So $\mathrm{Ker}d_{n}\otimes M$ is direct summands of $P_{n}\otimes M$, i.e. the direct summands of $\mathrm{Ker}(d_{n}\otimes id_{M})$. Quotient $\mathrm{Im}d_{n+1}\otimes M=\mathrm{Im}(d_{n+1}\otimes id_{M})$, we have $H_{n}(P)\otimes M$ to be the direct summands of $H_{n}(P\otimes M)$. ◻

Proof. Similar to universal coefficient theorem for homlogy. ◻

Proof. Similar to the proof for homology. ◻

Proof. Consider the exact sequence

$$0\to \mathrm{Ker}d_{i}^{C}\to C_{i}\to\mathrm{Im}d_{i}^{C}\to C$$

Apply fuctor $-\otimes D_{j}$ on it, we have

$$0\to \mathrm{Ker}d_{i}^{C}\otimes D_{j}\to C_{i}\otimes D_{j}\to \mathrm{Im}d_{i}^{C}\otimes D_{j}\to 0$$

To form a double chain complex, we define $d_{i}:\mathrm{Ker}d_{i}^{C}\to \mathrm{Ker}d_{i}^{C}$ and $d_{i}:\mathrm{Im}d_{i}^{C}\to \mathrm{Im}d_{i-1}^{C}$ to be the zero morphisms. So we have the exact sequence of tensors

$$0\to \mathrm{Ker}d^{C}\otimes D\to C\otimes D\to \mathrm{Im}d^{C}\otimes D\to 0$$

Use the long exact sequence theorem, we have the long exact sequence

$$\cdots\to H_{n+1}(\mathrm{Im}d^{C}\otimes D)\to H_{n}(\mathrm{Ker}d^{C}\otimes D)\to H_{n}(C\otimes D)\to H_{n}(\mathrm{Im}d^{C}\otimes D)\to \cdots$$

From the lemma above

$$\cdots\to \bigoplus_{i\in\mathbb{Z}} H_{n+1}(\mathrm{Im}d_{i}\otimes D_{\bullet -i})\to \bigoplus_{i\in \mathbb{Z}}H_{n}(\mathrm{Ker}d_{i}\otimes D_{\bullet -i})\xrightarrow{f} H_{n}(C\otimes D)\to \bigoplus_{i\in \mathbb{Z}}H_{n}(\mathrm{Im}d_{i}\otimes D_{\bullet -i})\xrightarrow{g}\bigoplus_{i\in \mathbb{Z}}H_{n-1}(\mathrm{Ker}d_{i}\otimes D_{\bullet -i})\to \cdots$$

Via shifting the complex

$$\left\{\begin{aligned} &\bigoplus_{i\in\mathbb{Z}}H_{n+1}(\mathrm{Im}d_{i}\otimes D_{\bullet -i})=\bigoplus_{i\in\mathbb{Z}}H_{n}(\mathrm{Im}d_{i}\otimes D_{\bullet +1-i})=\bigoplus_{i\in \mathbb{Z}}H_{n}(\mathrm{Im}d_{i+1}\otimes D_{\bullet -i})\\ &\bigoplus_{i\in\mathbb{Z}}H_{n-1}(\mathrm{Ker}d_{i}\otimes D_{\bullet -i})=\bigoplus_{i\in\mathbb{Z}}H_{n}(\mathrm{Ker}d_{i}\otimes D_{\bullet -1-i})=\bigoplus_{i\in \mathbb{Z}}H_{n}(\mathrm{Ker}d_{i-1}\otimes D_{\bullet -i})\end{aligned} \right.$$

So we have the long exact sequence

$$\cdots\to \bigoplus_{i\in\mathbb{Z}}H_{n}(\mathrm{Im}d_{i+1}\otimes D_{\bullet -i})\to \bigoplus_{i\in\mathbb{Z}}H_{n}(\mathrm{Ker}d_{i}\otimes D_{\bullet -i})\xrightarrow{f} H_{n}(C\otimes D)\to \bigoplus_{i\in\mathbb{Z}}H_{n}(\mathrm{Ker}d_{i}\otimes D_{\bullet -i})\xrightarrow{g}\bigoplus_{i\in \mathbb{Z}}H_{n}(\mathrm{Im}d_{i-1}\otimes D_{\bullet -i})\to \cdots$$

Consider the short exact sequence

$$0\to \mathrm{Im}f\to H_{n}(C\otimes D)\to\mathrm{Ker}g \to 0$$

We compute the short $\mathrm{Im}g$ and $\mathrm{Ker}g$. For the short exact sequence

$$0\to \mathrm{Im}d_{i+1}\to \mathrm{Ker}d_{i}\to H_{i}(C)\to 0$$

we have the long exact sequence

$$\cdots\to H_{n}(\mathrm{Im}d_{i+1}\otimes D_{\bullet -i})\to H_{n}(\mathrm{Ker}d_{i}\otimes d_{\bullet -i})\to H_{n}(H_{i}(C)\otimes D_{\bullet -i})\to \cdots$$

From the universal coefficient theorem

$$H_{n}(\mathrm{Im}d_{i+1}\otimes D_{\bullet -i})=\mathrm{Im}d_{i+1}\otimes H_{n}(D_{\bullet -i})$$

$$H_{n}(\mathrm{Ker}d_{i}\otimes D_{\bullet -i})= \mathrm{Ker}d_{i}\otimes H_{n}(D_{\bullet -i})$$

so

$$\begin{aligned} \mathrm{Im}f&=\bigoplus_{i\in\mathbb{Z}}\mathrm{Coker}(\mathrm{Im}d_{i+1}\otimes H_{n}(D_{\bullet -i})\to \mathrm{Ker}d_{i}\otimes H_{n}(D_{\bullet -i}))\\&=\bigoplus_{i\in\mathbb{Z}}H_{i}(C)\otimes H_{n}(D_{\bullet -i})\\&=\bigoplus_{i\in \mathbb{Z}}H_{i}(C)\otimes H_{n-i}(D)\end{aligned}$$

Similarly,

$$\begin{aligned} \mathrm{Ker}g&=\bigoplus_{i\in\mathbb{Z}}\mathrm{Ker}(\mathrm{Ker}d_{i-1}\otimes H_{n}(D_{\bullet -i})\to \mathrm{Im}d_{i}\otimes H_{n}(D_{\bullet -i}))\\&=\bigoplus_{i\in \mathbb{Z}}\mathrm{Tor}_{1}(H_{i-1}(C), H_{n}(D_{\bullet -i}))\\&=\bigoplus_{i\in\mathbb{Z}}\mathrm{Tor}_{1}(H_{i-1}(C), H_{n-i}(D))\end{aligned}$$

◻

Proof. Omitted. ◻